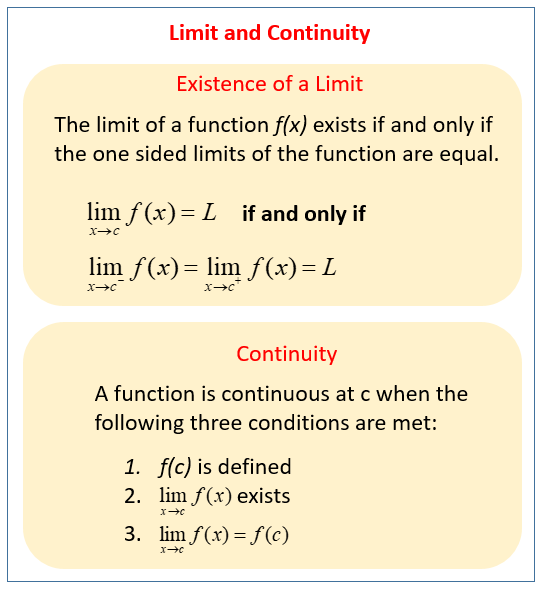
How To Determine Continuity From A Table at Olive Oconnell blog

How To Determine Continuity From A Table. If the function is discontinuous at −1, classify the discontinuity as removable, jump, or. The limit of the function. Given a piecewise function, determine whether it is continuous. We will also see the intermediate value. we begin our investigation of continuity by exploring what it means for a function to have continuity at a point. evaluating a limit using a table of functional values. Determine whether each component function of the piecewise function is continuous. we begin our investigation of continuity by exploring what it means for a function to have continuity at a point. To evaluate lim x → a f (x), lim x → a f (x), we begin by completing. continuity a function f of two variables is called continuous at (a;b) if lim (x;y)!(a;b) f(x;y) = f(a;b) i.e.  — in this section we will introduce the concept of continuity and how it relates to limits.  — courses on khan academy are always 100% free. determine whether \(f(x)=\frac{x+2}{x+1}\) is continuous at −1.

Determine whether each component function of the piecewise function is continuous. we begin our investigation of continuity by exploring what it means for a function to have continuity at a point. If the function is discontinuous at −1, classify the discontinuity as removable, jump, or. We will also see the intermediate value.  — courses on khan academy are always 100% free. evaluating a limit using a table of functional values. continuity a function f of two variables is called continuous at (a;b) if lim (x;y)!(a;b) f(x;y) = f(a;b) i.e. determine whether \(f(x)=\frac{x+2}{x+1}\) is continuous at −1. The limit of the function. we begin our investigation of continuity by exploring what it means for a function to have continuity at a point.

Calculus Limits Of Functions (video lessons, examples, solutions)

How To Determine Continuity From A Table The limit of the function. To evaluate lim x → a f (x), lim x → a f (x), we begin by completing. We will also see the intermediate value. determine whether \(f(x)=\frac{x+2}{x+1}\) is continuous at −1. If the function is discontinuous at −1, classify the discontinuity as removable, jump, or. Given a piecewise function, determine whether it is continuous. continuity a function f of two variables is called continuous at (a;b) if lim (x;y)!(a;b) f(x;y) = f(a;b) i.e.  — in this section we will introduce the concept of continuity and how it relates to limits.  — courses on khan academy are always 100% free. Determine whether each component function of the piecewise function is continuous. evaluating a limit using a table of functional values. we begin our investigation of continuity by exploring what it means for a function to have continuity at a point. The limit of the function. we begin our investigation of continuity by exploring what it means for a function to have continuity at a point.